
Clearly, the NCDB (2006) data revealed that, of the 9746 students in US schools who were reported to have deaf-blindness, 1743 (18%) had profound hearing loss 1401 (14%) had severe hearing loss 1074 (11%) had moderate-to-severe hearing loss 1332 (14%) had moderate hearing loss and 1423 (15%) had mild hearing loss. The 2006, NCDB deaf–blind census data reported on a range of hearing losses from mild (26–40 dB) to profound hearing loss (91 dB and above). Hearing loss is classified in degrees of hearing loss based on decibel levels of slight, ranging from 16 to 25 dB mild, ranging from 26 to 40 dB moderate, ranging from 41 to 50 dB and moderate-to-severe, ranging from 50 to 70 dB severe, ranging from 71 to 90 dB, and profound, ranging from 91 dB to worse ( Martin and Clark, 2000).
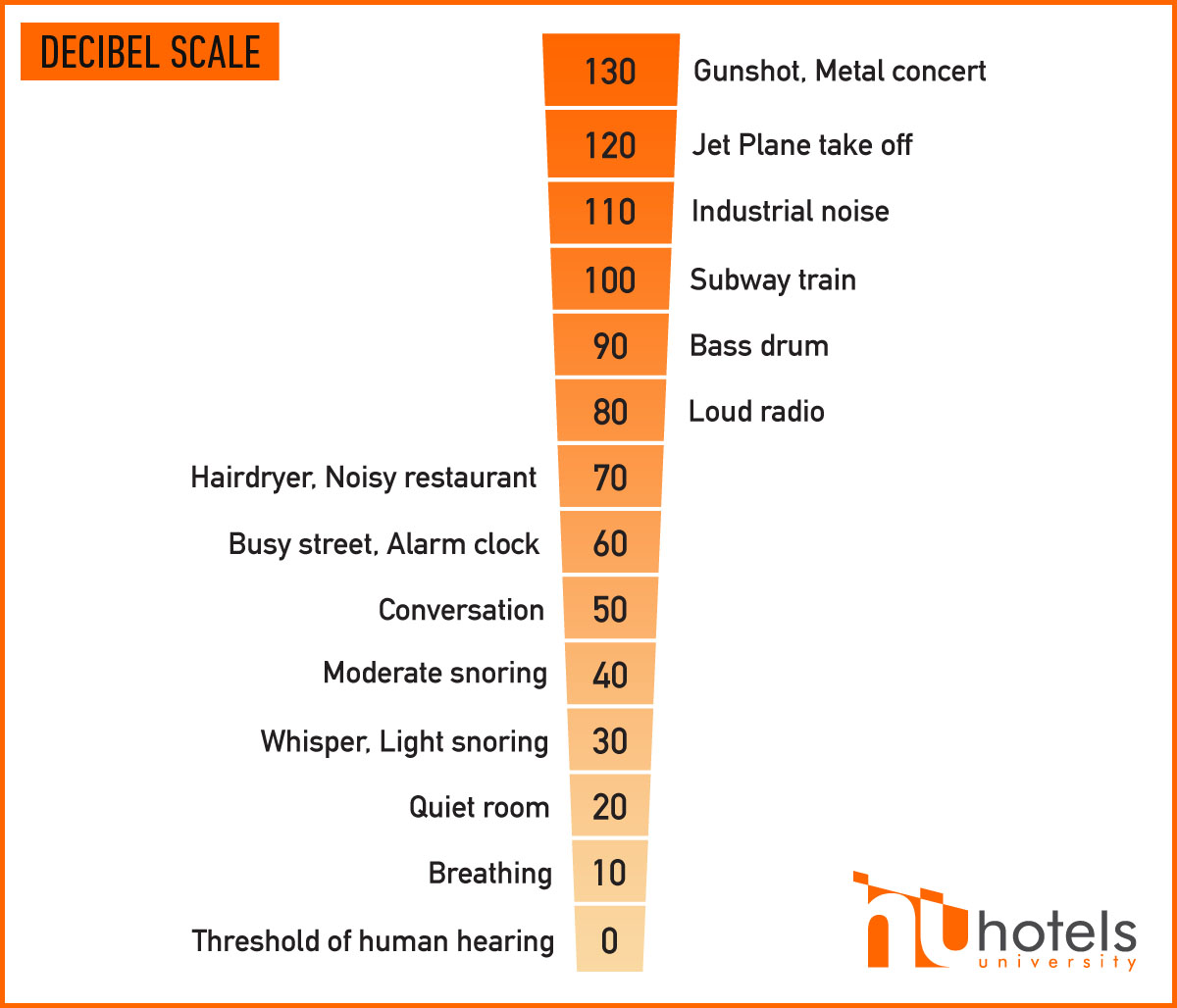
The frequencies used in audiological testing are in octave levels of 250, 500, 1000, 2000, 4000, and 8000 Hz ( Martin and Clark, 2000). The psychological perception or layman’s term for frequency is pitch. Hearing loss is documented by perception of loudness levels called decibels (dB) across frequency ranges or Hertz (Hz). Chinn, in International Encyclopedia of Education (Third Edition), 2010 Understanding Hearing Loss Substituting the value of a micropascal for the rms pressure in the plane wave intensity expression, we find that a plane wave pressure of 1 μ Pa corresponds to an intensity of 0.67 × 10 −22 W/cm 2 (i.e., 0 dB re 1 μ Pa). In seawater, ρ c is 1.5 × 10 5 g cm −2 s −1 so that a plane wave of rms pressure 1 dyne/cm 2 has an intensity of 0.67 × 10 −12 W/cm 2.

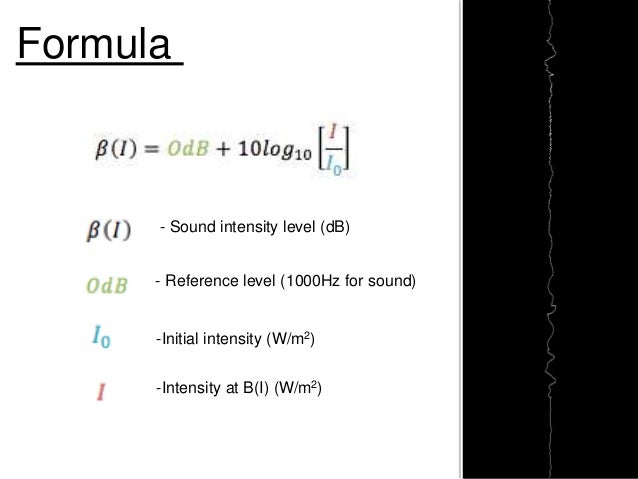
The average intensity, I, of a plane wave with rms pressure p in a medium of density ρ and sound speed c is I = p 2/ ρ c. Pressure ( p) ratios are expressed in dB re 1 μ Pa by taking 20 log p 1/ p 2 where it is understood that the reference originates from the intensity of a plane wave of pressure equal to 1 μ Pa.
Therefore, taking 1 μ Pa as I 2, a sound wave having an intensity, of, say, one million times that of a plane wave of rms pressure 1 μ Pa has a level of 10 log(10 6/1) ≡ 60 dB re 1 μ Pa. The presently accepted reference intensity is based on a reference pressure of one micropascal (μ Pa): the intensity of a plane wave having an rms pressurex equal to 10 −5 dynes per square centimeter. Absolute intensities can therefore be expressed by using a reference intensity. Two intensities, I 1 and I 2, have a ratio, I 1/ I 2, in decibels of 10 log I 1/ I 2 dB. The decibel (dB) is the dominant unit in underwater acoustics and denotes a ratio of intensities (not pressures) expressed in terms of a logarithmic (base 10) scale. Kuperman, in Encyclopedia of Physical Science and Technology (Third Edition), 2003 VIII Appendix: Units


 0 kommentar(er)
0 kommentar(er)
